BRENT ROBERTSON-Write up 11 Polar Equations
For this investigation I used the graphing calculator program to investigate some polar equations. As you know polar equations are given in terms of an angle theta and a distance from the origin of that point.
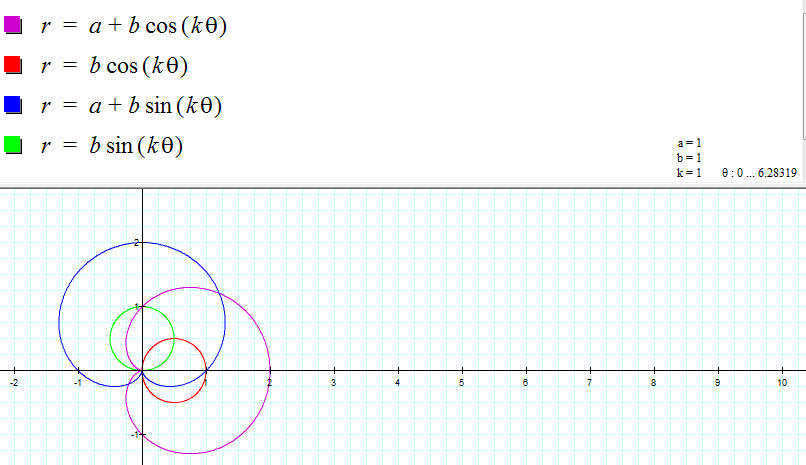
Cardiod pattern for the a plus b cos with symmetry on the x axis where y=0. For sin as expected symmetry on the y axis as described in write up number 10.
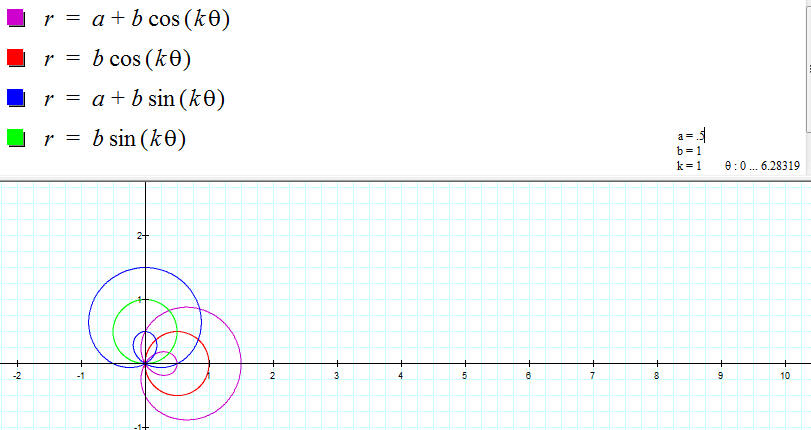
First we will manipulate a to a number between zero and one. One-half will do
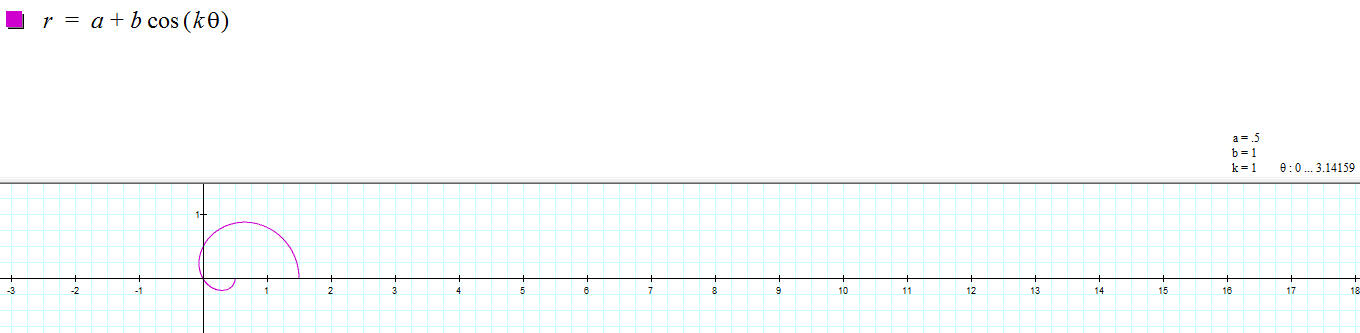
You can see the max x value for the cosine function is down to 1.5 from 2. From the perspective of the polar equation as the domain goes from zero to 2 pi, the polar output does in inverted figure eight. This pick-up pattern is also germaine to dynamic microphones such as the sure SM 57 where the cardiod graph represents the frequency response around the front of the microphone. Here is a look at the response through only 180 degrees of domain input.
Here is a half cycle with a>1
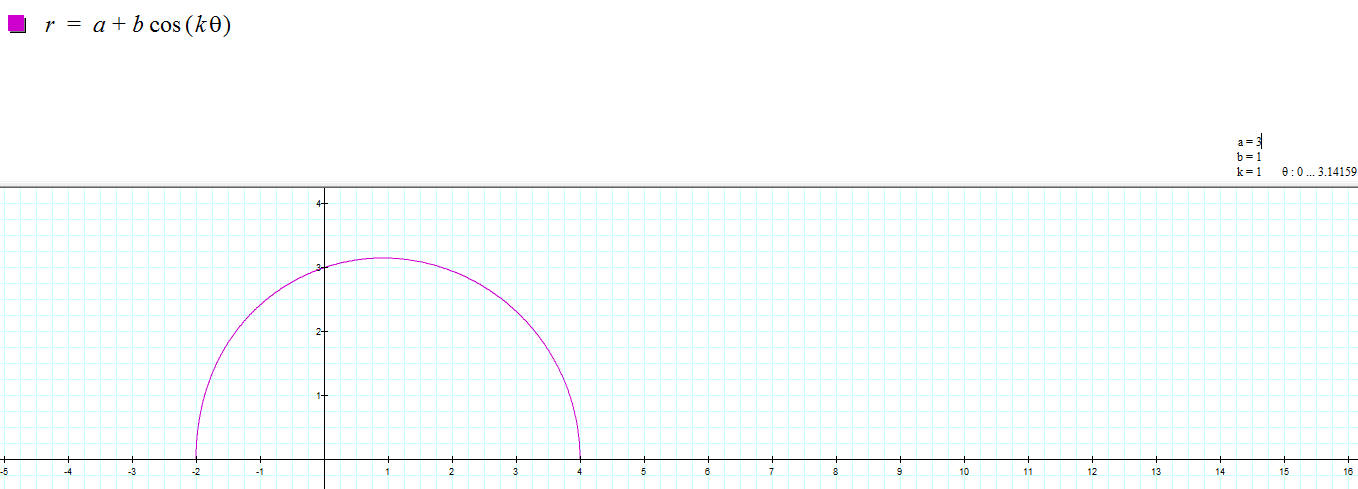
This show a semi-circle pattern with radius three, the CONSTANT we replaced for a.
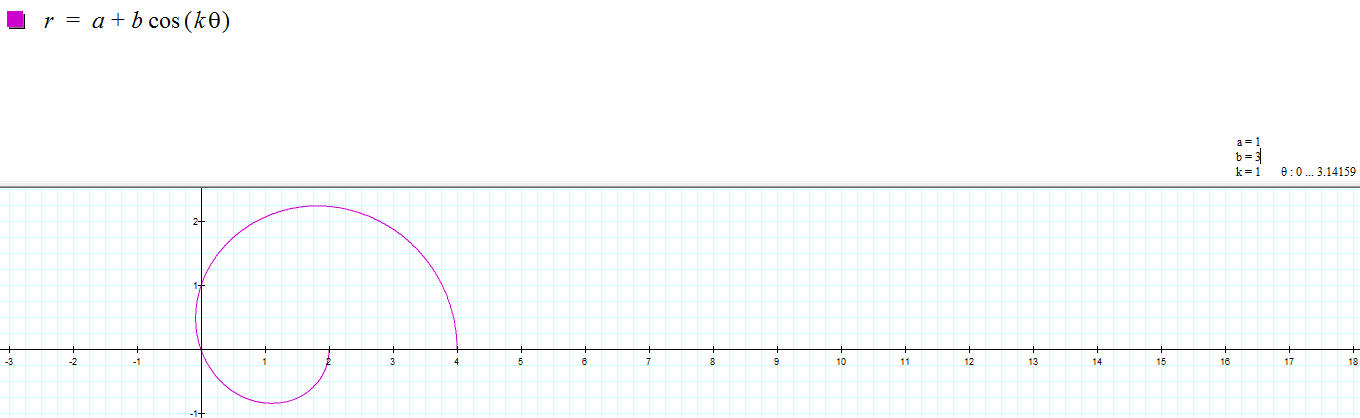
Now let's look at the manipulation of b. We will stay with a zero to pi domain knowing that we can reflect across the x axis to get the other half.
Here is b=.5

On this example the constant between 0 and 1 evens out the curve and inversely the value of b>1 creates the cardiod pattern associated with the SM-57 microphone.
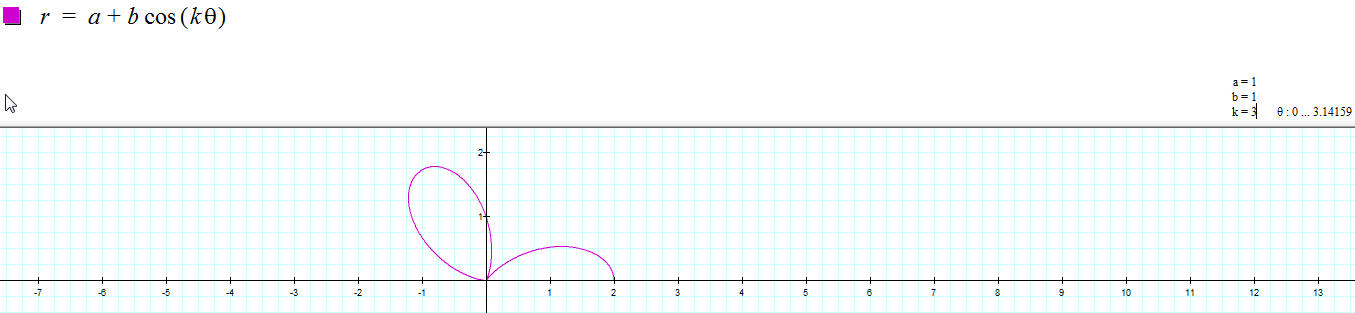
Here is the maniplation of k which is literally emeshed with the domain. This illustrates the paradigm difference between polar and rectanglular graphing. In repcangular x y graphing the manipulation of a term directly related to the input or domain will cause a horizontal shift in the opposite direction of the term. As a factor it will cause a vertical "stretch" or "compression" accordingly. Note what occurs in the Polar paradigm.
k=.5

Here is k>1